ISSN 0253-2778
CN 34-1054/N
By optimization methods on Riemannian submanifolds, we establish two inequalities between the intrinsic and extrinsic invariants, for generalized normalized δ-Casorati curvatures of warped product submanifolds in a Riemannian manifold of quasi-constant curvature. We generalize the conclusions of the optimal inequalities of submanifolds in real space forms.
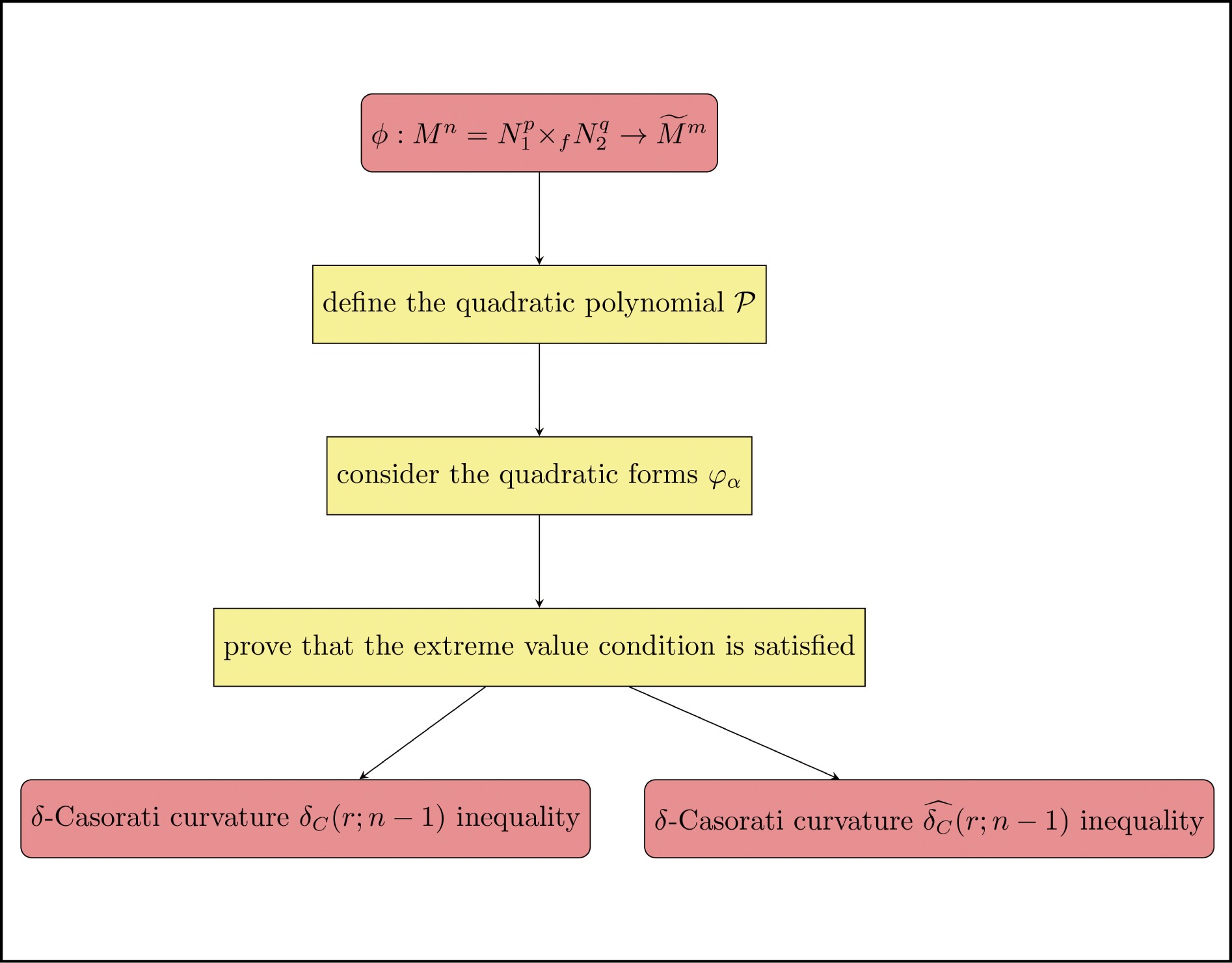
The process of establishing the generalized normalized δ-Casorati curvatures inequality.
| [1] |
Chen B Y. Some pinching and classification theorems for minimal submanifolds. Arch. Math., 1993, 60: 568–578. DOI: 10.1007/BF01236084
|
| [2] |
Chen B Y. A Riemannian invariant and its applications to submanifold theory. Results in Mathematics, 1995, 27: 17–26. DOI: 10.1007/BF03322265
|
| [3] |
Casorati F. Mesure de la courbure des surfaces suivant l'idée commune.: Ses rapports avec les mesures de courbure gaussienne et moyenne. Acta. Math., 1890, 14: 95–110. DOI: 10.1007/BF02413317
|
| [4] |
Decu S, Haesen S, Verstraelen L. Optimal inequalities involving Casorati curvatures. Bull.Transilv. Univ. Brasov Ser. B, 2007, 14: 85–93.
|
| [5] |
Decu S, Haesen S, Verstraelen L. Optimal inequalities characterising quasi-umbilical submanifolds. J. Inequal. Pure and Appl. Math., 2008, 9: 79.
|
| [6] |
Park K S. Inequalities for the Casorati curvatures of real hypersurfaces in some Grassmannians. Taiwanese J. Math., 2018, 22: 63–77. DOI: 10.11650/tjm/8124
|
| [7] |
Choudhary M A, Blaga A M. Inequalities for generalized normalized δ-Casorati curvatures of slant submanifolds in metallic Riemannian space forms. J. Geom., 2020, 111: 39. DOI: 10.1007/s00022-020-00552-5
|
| [8] |
Chen B Y, Yano K. Hypersurfaces of a conformally flat space. Tensor, N. S., 1972, 26: 318–322.
|
| [9] |
Chen B Y. Another general inequality for CR-warped products in complex space forms. Hokkaido Math. J., 2003, 32: 415–444.
|
| [10] |
Oprea T. Chen's inequality in the Lagrangian case. Colloq. Math., 2007, 108: 163–169. DOI: 10.4064/cm108-1-15
|
| [11] |
Vîlcu G E. An optimal inequality for Lagrangian submanifolds in complex space forms involving Casorati curvature. J. Math. Anal. Appl., 2018, 465: 1209–1222. DOI: 10.1016/j.jmaa.2018.05.060
|