| Materials | C | Si | Mn | P | S | Cu | Cr | Ni | Mo | Fe |
| Q355C | 0.17 | 0.55 | 1.60 | 0.03 | 0.03 | 0.40 | 0.30 | 0.30 | - | Bal. |
| E71T-1C | 0.18 | 0.90 | 1.75 | 0.03 | 0.03 | 0.35 | 0.20 | 0.50 | 0.30 | Bal. |
ISSN 0253-2778
CN 34-1054/N
Dirac’s theorem states that if a graph G on n vertices has a minimum degree of at least
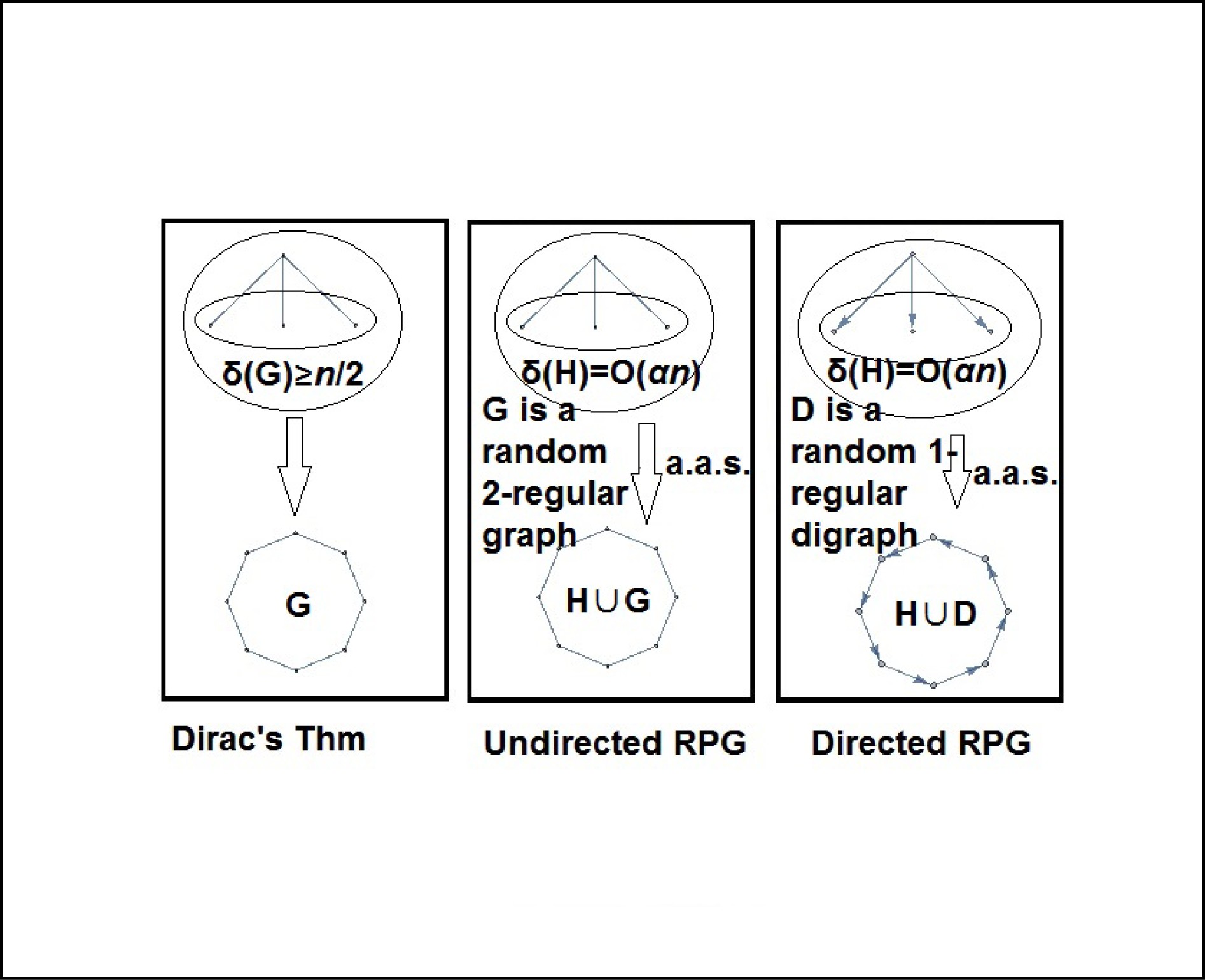
Minimum degree conditons on Hamilton cycles.
| [1] |
Dirac G A. Some theorems on abstract graphs. Proceedings of the London Mathematical Society, 1952, s3-2 (1): 69–81. DOI: 10.1112/plms/s3-2.1.69
|
| [2] |
Korsunov A D. Solution of a problem of Erdös and Rényi on Hamiltonian cycles in undirected graphs. Metody Diskret. Analiz., 1977, 31: 17–56.
|
| [3] |
Bohman T, Frieze A, Martin R. How many random edges make a dense graph Hamiltonian? Random Structures Algorithms, 2003, 22 (1): 33–42. DOI: 10.1002/rsa.10070
|
| [4] |
Krivelevich M, Kwan M, Sudakov B. Cycles and matchings in randomly perturbed digraphs and hypergraphs. Combinatorics, Probability and Computing, 2016, 25 (6): 909–927. DOI: 10.1017/S0963548316000079
|
| [5] |
Hahn-Klimroth M, Maesaka G S, Mogge Y, et al. Random perturbation of sparse graphs. The Electronic Journal of Combinatorics, 2021, 28 (2): P2.26. DOI: 10.37236/9510
|
| [6] |
Bedenknecht W, Han J, Kohayakawa Y, et al. Powers of tight Hamilton cycles in randomly perturbed hypergraphs. Random Structures & Algorithms, 2019, 55 (4): 795–807. DOI: 10.1002/rsa.20885
|
| [7] |
Han J, Zhao Y. Hamiltonicity in randomly perturbed hypergraphs. Journal of Combinatorial Theory, Series B, 2020, 144: 14–31. DOI: 10.1016/j.jctb.2019.12.005
|
| [8] |
Chang Y L, Han J, Thoma L. On powers of tight Hamilton cycles in randomly perturbed hypergraphs. https://doi.org/10.48550/ arXiv.2007.11775.
|
| [9] |
Condon P, Espuny Díaz A, Girão A, et al. Hamiltonicity of random subgraphs of the hypercube. In: Proceedings of the 2021 ACM-SIAM Symposium on Discrete Algorithms (SODA). Philadelphia, PA: SIAM, 2021.
|
| [10] |
Dudek A, Reiher C, Ruciński A, et al. Powers of Hamiltonian cycles in randomly augmented graphs. Random Structures & Algorithms, 2020, 56 (1): 122–141. DOI: 10.1002/rsa.20870
|
| [11] |
Böttcher J, Montgomery R, Parczyk O, et al. Embedding spanning bounded degree graphs in randomly perturbed graphs. Mathematika, 2020, 66 (2): 422–447. DOI: 10.1112/mtk.12005
|
| [12] |
Balogh J, Treglown A, Wagner A Z. Tilings in randomly perturbed dense graphs. Combinatorics, Probability and Computing, 2019, 28 (2): 159–176. DOI: 10.1017/S0963548318000366
|
| [13] |
Han J, Morris P, Treglown A. Tilings in randomly perturbed graphs: Bridging the gap between Hajnal-Szemerédi and Johansson-Kahn-Vu. Random Structures & Algorithms, 2021, 58 (3): 480–516. DOI: 10.1002/rsa.20981
|
| [14] |
Espuny Díaz A, Girão A, Hamiltonicity of graphs perturbed by a random regular graph. https://doi.org/10.48550/arXiv.2101.06689.
|
| [15] |
Cooper C, Frieze A, Molloy M. Hamilton cycles in random regular digraphs. Combinatorics, Probability and Computing, 1994, 3 (1): 39–49. DOI: 10.1017/S096354830000095X
|
| [16] |
Bollobás B. A probabilistic proof of an asymptotic formula for the number of labelled regular graphs. European Journal of Combinatorics, 1980, 1 (4): 311–316. DOI: 10.1016/S0195-6698(80)80030-8
|
| [17] |
Wormald N C. Models of random regular graphs. In: Surveys in Combinatorics. Cambridge, UK: Cambridge University Press, 1999.
|
| Materials | C | Si | Mn | P | S | Cu | Cr | Ni | Mo | Fe |
| Q355C | 0.17 | 0.55 | 1.60 | 0.03 | 0.03 | 0.40 | 0.30 | 0.30 | - | Bal. |
| E71T-1C | 0.18 | 0.90 | 1.75 | 0.03 | 0.03 | 0.35 | 0.20 | 0.50 | 0.30 | Bal. |
| Factors | Notation | Unit | Levels | |||||
| 1 | 2 | 3 | 4 | 5 | 6 | |||
| Preheating temperature | T | ℃ | 25 | 60 | 120 | 180 | 240 | 300 |
| Welding voltage | U | V | 20 | 26 | 32 | |||
| Welding current | I | A | 180 | 220 | 260 | |||
| Welding speed | S | cm·min−1 | 22 | 27 | 32 | |||
| Wire extension length | L | mm | 17 | 21 | 25 | |||
| Run | Preheating temperature (℃) | Welding voltage (V) | Welding current (A) | Welding speed (cm·min−1) | Wire extension length (mm) | Aspect ratio | Hardness (HV) | Residual stress (MPa) |
| 1 | 25 | 20 | 180 | 22 | 17 | 0.791 | 243.8 | 141.23 |
| 2 | 25 | 26 | 220 | 27 | 21 | 0.372 | 210.6 | 40.81 |
| 3 | 25 | 32 | 260 | 32 | 25 | 0.272 | 210.0 | 53.51 |
| 4 | 60 | 20 | 180 | 27 | 25 | 0.957 | 250.4 | 84.62 |
| 5 | 60 | 26 | 220 | 32 | 17 | 0.481 | 228.8 | 61.92 |
| 6 | 60 | 32 | 260 | 22 | 21 | 0.111 | 205.4 | 64.83 |
| 7 | 120 | 20 | 220 | 32 | 25 | 0.970 | 234.3 | 120.83 |
| 8 | 120 | 26 | 260 | 22 | 17 | 0.445 | 225.5 | 91.98 |
| 9 | 120 | 32 | 180 | 27 | 21 | 0.264 | 216.9 | 54.22 |
| 10 | 180 | 20 | 260 | 27 | 17 | 0.680 | 236.3 | 47.63 |
| 11 | 180 | 26 | 180 | 32 | 21 | 0.258 | 230.0 | 79.87 |
| 12 | 180 | 32 | 220 | 22 | 25 | 0.202 | 221.1 | 145.89 |
| 13 | 240 | 20 | 220 | 22 | 21 | 0.992 | 231.6 | 123.59 |
| 14 | 240 | 26 | 260 | 27 | 25 | 0.401 | 249.5 | 65.90 |
| 15 | 240 | 32 | 180 | 32 | 17 | 0.228 | 213.3 | 125.39 |
| 16 | 300 | 20 | 260 | 32 | 21 | 0.960 | 267.9 | 68.37 |
| 17 | 300 | 26 | 180 | 22 | 25 | 0.622 | 235.9 | 148.50 |
| 18 | 300 | 32 | 220 | 27 | 17 | 0.197 | 251.5 | 148.86 |
| Project | Source | DF | Seq. SS | Adj. MS | F value | p value | Contribution |
| Aspect ratio | Regression | 6 | 6.1376 | 1.02293 | 67.26 | <0.001 | 97.11% |
| T | 1 | 3.5686 | 0.00949 | 0.62 | 0.445 | 56.46% | |
| U | 1 | 0.3788 | 1.38479 | 91.05 | <0.001 | 5.99% | |
| I | 1 | 1.1641 | 0.42758 | 28.11 | <0.001 | 18.42% | |
| S | 1 | 0.3611 | 0.00004 | 0.01 | 0.958 | 5.71% | |
| L | 1 | 0.3040 | 0.03220 | 2.12 | 0.171 | 4.81% | |
| I*I | 1 | 0.3611 | 0.36112 | 23.74 | <0.001 | 5.71% | |
| Error | 12 | 0.1825 | 0.01521 | - | - | 2.89% | |
| Total | 18 | 6.3201 | - | - | - | 100.00% | |
| R2 | 97.11% | R2(adjusted) | 95.67% | R2(predicted) | 93.92% | ||
| Hardness | Regression | 9 | 4798.48 | 533.16 | 49.24 | <0.001 | 98.23% |
| T | 1 | 1183.46 | 798.41 | 73.74 | <0.001 | 24.23% | |
| U | 1 | 1778.77 | 756.19 | 69.84 | <0.001 | 36.41% | |
| I | 1 | 10.37 | 645.70 | 59.63 | <0.001 | 0.21% | |
| S | 1 | 50.14 | 139.02 | 12.84 | 0.007 | 1.03% | |
| L | 1 | 0.06 | 226.71 | 20.94 | 0.002 | 0.01% | |
| T*I | 1 | 860.11 | 1173.40 | 108.37 | <0.001 | 17.61% | |
| U*I | 1 | 583.39 | 709.59 | 65.54 | <0.001 | 11.94% | |
| I*S | 1 | 88.88 | 217.59 | 20.10 | 0.002 | 1.82% | |
| I*L | 1 | 243.30 | 243.30 | 22.47 | 0.001 | 4.98% | |
| Error | 8 | 86.62 | 10.83 | - | - | 1.77% | |
| Total | 17 | 4885.10 | - | - | - | 100.00% | |
| R2 | 98.23% | R2(adjusted) | 96.23% | R2(predicted) | 87.82% | ||
| Residual stress | Regression | 9 | 24526.0 | 2725.11 | 29.26 | <0.001 | 97.05% |
| T | 1 | 4655.8 | 1365.90 | 14.66 | 0.005 | 18.42% | |
| U | 1 | 3.4 | 2429.34 | 26.08 | 0.001 | 0.01% | |
| I | 1 | 5021.7 | 2.37 | 0.03 | 0.877 | 19.87% | |
| S | 1 | 2675.5 | 5724.48 | 61.46 | <0.001 | 10.59% | |
| L | 1 | 166.7 | 281.65 | 3.02 | 0.120 | 0.66% | |
| U*U | 1 | 2447.9 | 1393.48 | 14.96 | 0.005 | 9.69% | |
| S*S | 1 | 1851.4 | 5672.95 | 60.90 | <0.001 | 7.33% | |
| T*U | 1 | 7165.1 | 7500.58 | 80.52 | <0.001 | 28.35% | |
| T*S | 1 | 538.5 | 538.50 | 5.78 | 0.043 | 2.13% | |
| Error | 8 | 745.2 | 93.15 | - | - | 2.95% | |
| Total | 17 | 25271.2 | - | - | - | 100.0% | |
| R2 | 97.05% | R2(adjusted) | 93.73% | R2(predicted) | 83.68% |
| Population quantity | Maximum iterations | Display scale of the noninferior solution | Function tolerance |
| 100 | 200 | 0.03 | 10×10−10 |
| Run | T (℃) | U (V) | I (A) | S (cm·min−1) | L (mm) | Aspect ratio | Hardness (HV) | Residual stress (MPa) |
| 1 | 28.02 | 31.93 | 256.62 | 27.87 | 17.16 | 0.093 | 218.96 | 0.09 |
| 2 | 33.90 | 20.57 | 259.19 | 22.44 | 24.71 | 0.823 | 165.69 | 130.88 |
| 3 | 34.15 | 22.96 | 258.84 | 23.43 | 24.73 | 0.690 | 173.69 | 87.43 |
| 4 | 38.42 | 32.00 | 259.74 | 30.72 | 17.03 | 0.081 | 230.04 | 25.84 |
| 5 | 34.35 | 21.44 | 258.82 | 22.30 | 24.66 | 0.774 | 167.78 | 121.89 |
| 6 | 33.87 | 20.15 | 259.81 | 22.24 | 24.90 | 0.847 | 163.18 | 140.21 |
| 7 | 37.50 | 32.00 | 259.13 | 28.20 | 17.03 | 0.082 | 223.05 | 5.70 |
| 8 | 33.98 | 24.13 | 258.70 | 22.90 | 24.89 | 0.626 | 174.46 | 83.60 |
| 9 | 34.14 | 29.08 | 258.50 | 25.38 | 23.69 | 0.333 | 193.99 | 23.38 |
| 10 | 33.68 | 31.97 | 258.75 | 28.19 | 17.03 | 0.084 | 221.86 | 3.73 |
| 11 | 35.32 | 22.70 | 259.32 | 22.44 | 24.66 | 0.702 | 170.58 | 105.03 |
| 12 | 32.46 | 21.92 | 257.82 | 22.69 | 25.00 | 0.754 | 169.53 | 110.09 |
| 13 | 34.33 | 25.14 | 258.18 | 22.20 | 24.26 | 0.562 | 176.63 | 85.26 |
| 14 | 33.99 | 21.44 | 258.12 | 22.54 | 24.83 | 0.779 | 168.60 | 118.21 |
| 15 | 28.77 | 31.38 | 256.67 | 27.00 | 18.60 | 0.142 | 212.80 | 2.33 |
| 16 | 33.93 | 21.33 | 258.34 | 22.76 | 24.85 | 0.784 | 168.69 | 115.98 |
| 17 | 34.38 | 25.42 | 258.21 | 22.80 | 24.11 | 0.545 | 179.03 | 73.15 |
| 18 | 34.13 | 23.26 | 258.19 | 22.47 | 24.74 | 0.675 | 172.33 | 98.65 |
| 19 | 34.35 | 20.91 | 259.36 | 22.97 | 24.66 | 0.803 | 167.89 | 117.87 |
| 20 | 33.59 | 26.17 | 259.19 | 22.71 | 23.44 | 0.490 | 180.99 | 67.78 |
| T (℃) | U (V) | I (A) | S (cm·min−1) | L (mm) | Aspect ratio | Hardness (HV) | Residual stress (MPa) | |
| Predict | 34.14 | 29.08 | 258.50 | 25.38 | 23.69 | 0.333 | 193.99 | 23.38 |
| Experimental | 34 | 29 | 259 | 25 | 24 | 0.302 | 204.2 | 24.12 |
| Error | 9.30% | 5.00% | 3.07% |